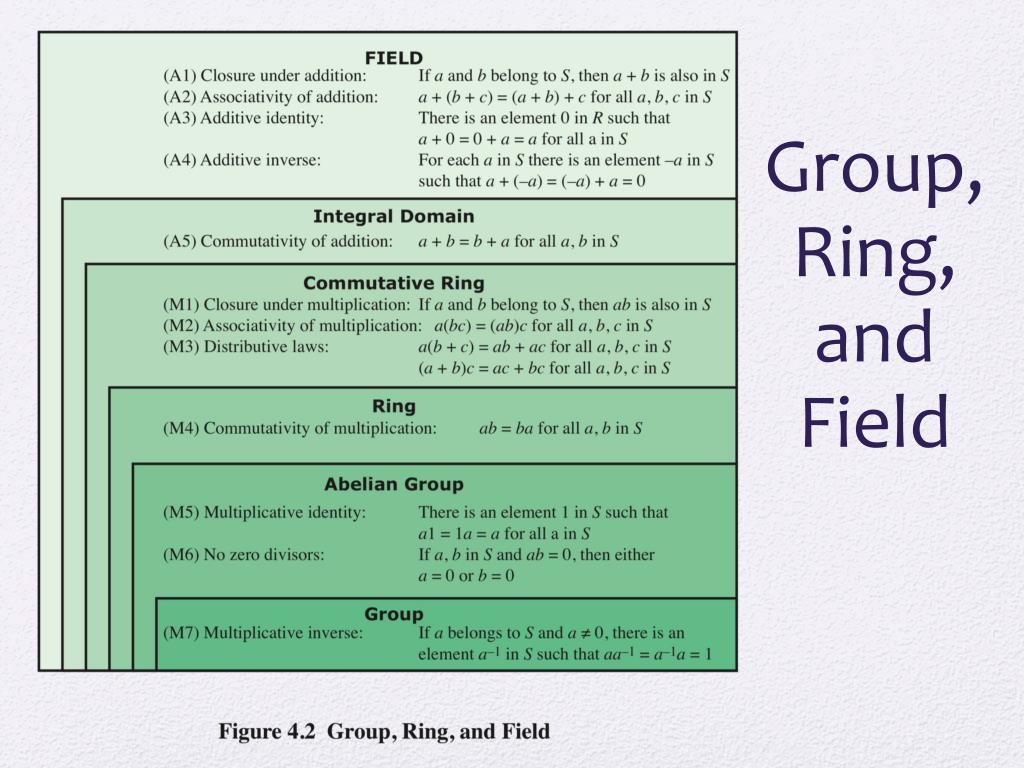
Ring Vs Field . Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Web a field is a set f which is closed under two operations + and × such that (1) f is an abelian group under + and (2) f −{0} (the.
from netgroup.edu.vn
Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Web a field is a set f which is closed under two operations + and × such that (1) f is an abelian group under + and (2) f −{0} (the. Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in.
Discover more than 143 group ring field in cryptography latest
Ring Vs Field Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Web a field is a set f which is closed under two operations + and × such that (1) f is an abelian group under + and (2) f −{0} (the.
From www.mathcounterexamples.net
Infinite rings and fields with positive characteristic Math Ring Vs Field Web a field is a set f which is closed under two operations + and × such that (1) f is an abelian group under + and (2) f −{0} (the. Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Rings in the previous section, we observed that many familiar number. Ring Vs Field.
From www.victoriana.com
unzureichend Hampelmann Th groups rings and fields Pop Motor Qualifikation Ring Vs Field Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Web every field is a ring, and the concept of a ring can be thought of as. Ring Vs Field.
From www.youtube.com
302.10B Fields as Quotients of Rings YouTube Ring Vs Field Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web every. Ring Vs Field.
From www.chegg.com
Solved Find an expression for the magnitude of the electric Ring Vs Field Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Web. Ring Vs Field.
From medschool.co
Peripheral Visual Fields Cranial Nerves MedSchool Ring Vs Field Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Web a field is a set f which is closed under two operations + and × such that (1) f is an abelian group under + and (2) f −{0} (the. Web a field is. Ring Vs Field.
From www.slideserve.com
PPT PART I Symmetric Ciphers CHAPTER 4 Finite Fields 4.1 Groups Ring Vs Field Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Web a. Ring Vs Field.
From www.slideserve.com
PPT Rings and fields PowerPoint Presentation, free download ID2062483 Ring Vs Field Web a field is a set f which is closed under two operations + and × such that (1) f is an abelian group under + and (2) f −{0} (the. Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Rings in the previous. Ring Vs Field.
From www.slideserve.com
PPT Network Coding AAU Summer School Finite Fields PowerPoint Ring Vs Field Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Web a. Ring Vs Field.
From www.slideserve.com
PPT Rings and fields PowerPoint Presentation, free download ID2872841 Ring Vs Field Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web a field is a set f which. Ring Vs Field.
From www.victoriana.com
unzureichend Hampelmann Th groups rings and fields Pop Motor Qualifikation Ring Vs Field Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Web a field is a set f which is closed under two operations + and × such that (1) f is an abelian group under + and (2) f −{0} (the. Web every field is a ring, and the concept of a ring can be. Ring Vs Field.
From www.youtube.com
Network Security and Cryptography Algebraic Structures Groups, Rings Ring Vs Field Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Web a field is a set f which is closed under two operations + and ×. Ring Vs Field.
From www.youtube.com
Field Court Pitch Track Ring YouTube Ring Vs Field Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Web a field is a set f which is closed under. Ring Vs Field.
From byjus.com
1.What is the electric field vs radius graph in a ring? Ring Vs Field Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Rings in the. Ring Vs Field.
From www.slideserve.com
PPT Cryptography and Network Security Chapter 4 PowerPoint Ring Vs Field Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Web a field is a set f which is closed under two operations + and × such that (1) f is an abelian group under + and (2) f −{0} (the. Web every field is a. Ring Vs Field.
From www.youtube.com
Rings, Fields and Finite Fields YouTube Ring Vs Field Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web. Ring Vs Field.
From www.slideserve.com
PPT Rings,Fields PowerPoint Presentation, free download ID680761 Ring Vs Field Web a field is a ring where the multiplication is commutative and every nonzero element has a multiplicative. Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Web a field is a set f which is closed under two operations + and × such that. Ring Vs Field.
From www.youtube.com
RNT2.5. Polynomial Rings over Fields YouTube Ring Vs Field Web every field is a ring, and the concept of a ring can be thought of as a generalisation of the concept of a field. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Web. Ring Vs Field.
From www.youtube.com
RINGS AND FIELDS DEFINITION YouTube Ring Vs Field Web a polynomial ring \(r[x]\) over a ring \(r\) is defined as \(\{(p(x)=a_0+a_1x+\cdots+a_nx^n| n \in. Web the structures similar to the set of integers are called rings, and those similar to the set of real numbers are called fields. Rings in the previous section, we observed that many familiar number systems are fields but that some are not. Web every. Ring Vs Field.